You signed in with another tab or window. Reload to refresh your session.You signed out in another tab or window. Reload to refresh your session.You switched accounts on another tab or window. Reload to refresh your session.Dismiss alert
I am little bit confused after reading your paper. Please correct me if I misunderstood.
In your paper, you show difference between VAE and WAE in terms of distribution matching objective
VAE: matching Q(z|x) to P(z)
WAE: matching Q(z) directly to P(z)
However, I wonder why you implemented WAE that matches Q(z|x) to P(z) with GAN and MMD.
(ex. Discriminator still discriminates z tilda from Q(z|x) and z from P(z)
In order to match Q(z) to P(z), don't you have to calculate distance between Q(z) and P(z), in which Q(z) is obtained by marginalizing Q(z|x) with P(x)?
But you are averaging distance between Q(z|x) and P(z) with multiple x.
The text was updated successfully, but these errors were encountered:
thanks for the question. When you sample $X_1, ..., X_n$ from the data distribution $P$ and then for every $i=1,...,n$ sample $Z_i$ from $Q(Z|X_i)$ then the resulting $Z_1,\dots,Z_n$ is a sample of independent points each one distributed according to the aggregate posterior $Q_Z$. Thus we are matching $Q_Z$ to $P_Z$ based on two samples: one from $Q_Z$ and one from $P_Z$.
Hi,
I am little bit confused after reading your paper. Please correct me if I misunderstood.
In your paper, you show difference between VAE and WAE in terms of distribution matching objective
However, I wonder why you implemented WAE that matches Q(z|x) to P(z) with GAN and MMD.
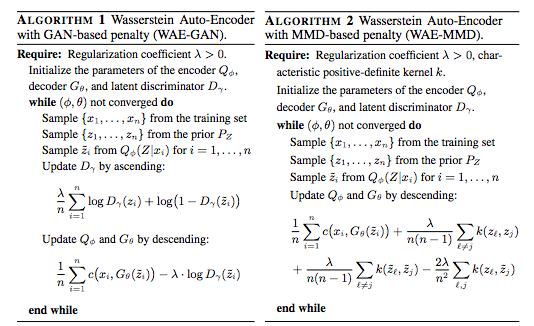
(ex. Discriminator still discriminates z tilda from Q(z|x) and z from P(z)
In order to match Q(z) to P(z), don't you have to calculate distance between Q(z) and P(z), in which Q(z) is obtained by marginalizing Q(z|x) with P(x)?
But you are averaging distance between Q(z|x) and P(z) with multiple x.
The text was updated successfully, but these errors were encountered: